Geometry is a fascinating subject, providing a visual and tangible way to understand spatial relationships, angles, and shapes. It can be a challenging subject for some, however, and understanding the basics of geometry can be a great way to get started. One of the most important concepts in geometry is understanding the term “converse.” This article will provide an overview of what converse means in geometry, as well as provide examples of how to use it in problem-solving. So, if you are looking to get a better understanding of converse in geometry, read on!
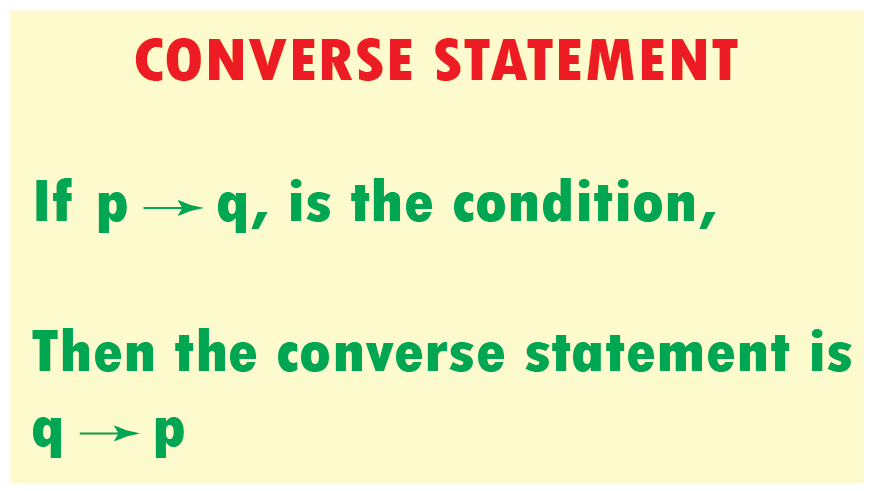
In geometry, converse means to switch the order of two statements in a theorem or proposition and see if the resulting statement is still true. For example, the theorem “If two angles are supplementary, then they add up to 180 degrees” can be switched to “If two angles add up to 180 degrees, then they are supplementary”. This is known as the converse of the theorem.
Converse in Geometry: Understanding the Definition
Converse in geometry is a term that is used to describe the reverse of a given statement. In geometry, a converse statement is created when the hypothesis and conclusion of a statement are interchanged. This is done to check the validity of the statement and to determine whether the two statements are logically equivalent.
For example, if the statement is “If it is raining, then the ground is wet”, the converse of this statement would be “If the ground is wet, then it is raining.” In this case, the converse statement would be true if it is raining, but false if it is not raining.
In addition to testing the validity of a statement, converse in geometry can also be used to determine the relationships between two different items or ideas. For example, if the statement is “If two angles are congruent, then they have the same measure”, the converse of this statement would be “If two angles have the same measure, then they are congruent.”
What is the difference between a Converse Statement and an Inverse Statement?
The difference between a converse statement and an inverse statement is that a converse statement involves switching the hypothesis and conclusion of a given statement while an inverse statement involves negating both the hypothesis and the conclusion. For example, the inverse of the statement “If it is raining, then the ground is wet” would be “If it is not raining, then the ground is not wet.”
Inverse statements can also be used to check the validity of a statement. For example, if the statement is “If two angles are congruent, then they have the same measure”, the inverse of this statement would be “If two angles do not have the same measure, then they are not congruent.” This inverse statement would be true if the two angles do not have the same measure, but false if they do have the same measure.
What is the importance of Converse in Geometry?
The importance of converse in geometry is that it allows us to test the validity of a statement and to determine the relationships between two different items or ideas. It also allows us to make logical deductions and to gain a better understanding of the properties of geometric figures.
For example, if we know that the converse of a statement is true, then we can deduce that the original statement is also true. This can be useful in proving theorems in geometry, as it allows us to make logical deductions from a given statement.
How can Converse be used in Problem Solving?
Converse can be used to solve problems in geometry by testing the validity of a statement and by deducing relationships between two different items or ideas. For example, if we know that the converse of a given statement is true, then we can deduce that the original statement is also true. This can be useful in problem solving as it allows us to make logical deductions and to gain a better understanding of the properties of geometric figures.
How is Converse used in Real-World Applications?
Converse can be used in real-world applications to test the validity of a statement and to determine the relationships between two different items or ideas. For example, it can be used to check the validity of a contract or agreement, to make logical deductions based on a given statement, or to gain a better understanding of the properties of a physical object or system.
It can also be used to test the validity of scientific theories and to make logical deductions from a given set of data. In addition, it can be used to make decisions based on a given set of facts or to determine the relationships between different items or ideas.
Related Faq
What Does Converse Mean in Geometry?
Answer: In geometry, the term converse is used to refer to the opposite of a statement or theorem. A converse statement is formed by switching the hypothesis and conclusion of a given statement. For example, the converse of the statement “If two angles are equal, then they are supplementary” is “If two angles are supplementary, then they are equal”. A converse statement is not necessarily true and must be proven in order to be accepted.
What Is an Example of a Converse Statement?
Answer: An example of a converse statement is “If two angles are supplementary, then they are equal”. This statement is formed by switching the hypothesis and conclusion of the statement “If two angles are equal, then they are supplementary”.
What Is the Difference Between a Converse Statement and an Inverse Statement?
Answer: The difference between a converse statement and an inverse statement is that a converse statement is formed by switching the hypothesis and conclusion of a given statement, while an inverse statement is formed by negating both the hypothesis and conclusion of a given statement. For example, the inverse of the statement “If two angles are equal, then they are supplementary” is “If two angles are not equal, then they are not supplementary”.
Does a Converse Statement Have to Be True?
Answer: No, a converse statement does not have to be true. A converse statement must be proven in order to be accepted as true. For example, the converse statement “If two angles are supplementary, then they are equal” must be proven in order to be accepted as true.
What Is a Contrapositive Statement?
Answer: A contrapositive statement is a statement formed by taking the contrapositive of a given statement. The contrapositive of a statement is formed by negating both the hypothesis and conclusion of the statement, and then switching the hypothesis and conclusion. For example, the contrapositive of the statement “If two angles are equal, then they are supplementary” is “If two angles are not supplementary, then they are not equal”.
How Do You Prove a Converse Statement?
Answer: To prove a converse statement, you must use the same logic and proof techniques used to prove any other statement. You must start by assuming that the hypothesis of the converse statement is true, and then use this assumption to logically prove that the conclusion of the statement is also true. For example, to prove the converse statement “If two angles are supplementary, then they are equal”, you would start by assuming that the two angles are supplementary, and then use this assumption to prove that the two angles are equal.
Converse, Inverse, & Contrapositive – Conditional & Biconditional Statements, Logic, Geometry
In conclusion, it can be seen that converse in geometry is a powerful concept that allows for the evaluation of two given statements and determining the truth of their relationship. It is an important tool in understanding geometric relationships and can be used to solve many problems. Converse in geometry is a critical skill for anyone looking to understand the nature of geometric relationships.
