Math is one of the most important and fundamental tools we have to help us understand the world around us. But what does “converse” mean when it comes to math? In this article, we’ll explore the concept of converse in mathematics, looking at its definition, its uses, and its implications. We’ll also discuss some examples of how converse is used in different types of math, as well as its importance to our understanding of the world and its implications for the future. So let’s get started and find out what converse means in math!
In mathematics, converse means to switch the order of two statements in a logical argument. For example, the statement “If a number is even, then it is divisible by 2” can be flipped to create its converse, which is “If a number is divisible by 2, then it is even.” In order for two statements to be considered converses, they must have the same truth value. That is, if one statement is true, then the other must also be true, and if one is false, the other must also be false. For example, the statement “If it is raining, then the ground is wet” is true, and its converse, “If the ground is wet, then it is raining,” is also true.
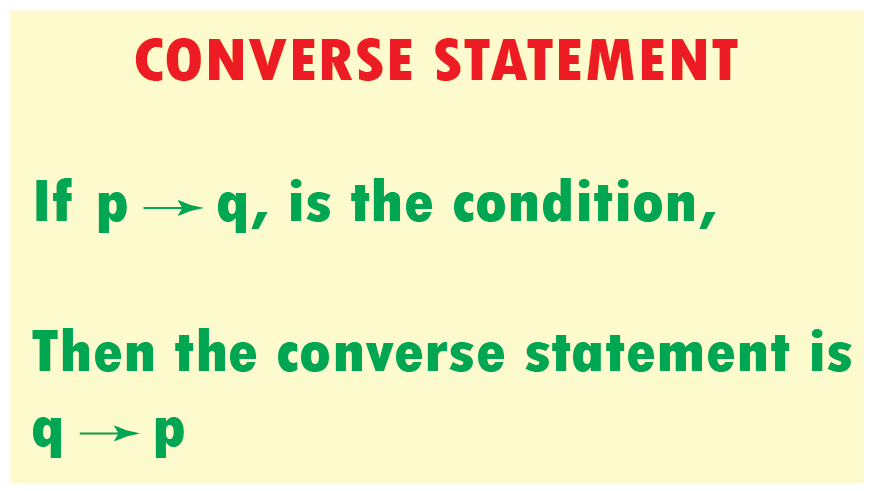
What Does Converse Mean in Mathematics?
Converse in mathematics is a way of expressing the inverse relationship between two statements. In mathematics, converse can also be used to describe the relationship between two statements, where one statement implies the other, or vice versa. Converse is a type of logical statement in which two statements are related to each other, and the truth of one depends on the truth of the other. It is important to understand the concept of converse in mathematics in order to be able to understand the implications of different mathematical statements.
In mathematics, a converse statement is one in which the truth of one statement depends on the truth of the other statement. For example, if statement A is true, then statement B must also be true. This is known as a converse statement. The converse of A is B, and the converse of B is A. The relationship between these two statements is known as the converse relationship.
Examples of Converse in Mathematics
Converse is used in many different areas of mathematics. For example, in geometry, the converse of the Pythagorean theorem states that if the sum of the squares of the lengths of the two sides of a right triangle is equal to the square of the length of the hypotenuse, then the triangle is a right triangle. Similarly, the converse of the triangle inequality theorem states that if the sum of the lengths of any two sides of a triangle is greater than the length of the third side, then the triangle is not a right triangle.
In algebra, the converse of a linear equation states that if two variables are related by a linear equation, then the equation is satisfied if and only if the two variables are equal. Similarly, the converse of a quadratic equation states that if two variables are related by a quadratic equation, then the equation is satisfied if and only if the two variables are equal.
Converse Statements in Logic
In logic, a converse statement is one in which the truth of the statement is dependent on the truth of the other statement. For example, if statement A is true, then statement B must also be true. This is known as a converse statement. The converse of A is B, and the converse of B is A. The relationship between these two statements is known as the converse relationship.
In logic, converse statements are used to determine if two statements are logically equivalent. For example, if statement A is true, then statement B must also be true. If statement B is true, then statement A must also be true. This means that the two statements are logically equivalent and that they are interchangeable.
Conditional Statements and Converse Statements
In logic, a conditional statement is one in which the truth of the statement is dependent on the truth of the other statement. For example, if statement A is true, then statement B must also be true. This is known as a conditional statement. The converse of a conditional statement is the inverse of the statement, which is statement B.
For example, if statement A is true, then statement B must also be true. The converse of this statement is if statement B is true, then statement A must also be true. This means that the two statements are logically equivalent and that they are interchangeable.
Types of Converse Statements
There are two types of converse statements in logic: direct and indirect. A direct converse statement is one in which the truth of the statement is dependent on the truth of the other statement. For example, if statement A is true, then statement B must also be true.
An indirect converse statement is one in which the truth of the statement is dependent on the truth of the other statement, but the statements are not logically equivalent. For example, if statement A is true, then statement B must also be true. However, if statement B is true, then statement A may not be true. This means that the two statements are not logically equivalent and that they are not interchangeable.
Conclusion
In conclusion, converse in mathematics is a way of expressing the inverse relationship between two statements. It is important to understand the concept of converse in order to be able to understand the implications of different mathematical statements. Examples of converse statements in mathematics include the Pythagorean theorem and the triangle inequality theorem. In logic, converse statements are used to determine if two statements are logically equivalent. There are two types of converse statements: direct and indirect.
Frequently Asked Questions
What Does Converse Mean in Math?
Answer: In mathematics, the converse of a statement is the inverse of the statement. That is, the converse is a statement formed by reversing the order of the two parts of the original statement. For example, if the statement is “If x is greater than y, then y is less than x,” then the converse of the statement would be “If y is less than x, then x is greater than y.” It is important to note that the converse of a statement may not be true, even if the original statement is true.
What Are the Different Types of Converse Statements?
Answer: There are two types of converse statements: conditional statements and biconditional statements. A conditional statement is one in which one part is true and the other part is false. For example, “If x is greater than y, then y is less than x” is a conditional statement. A biconditional statement is one in which both parts are true or both parts are false. For example, “If x is greater than y, then y is not greater than x” is a biconditional statement.
What Are Examples of Converse Statements?
Answer: Examples of converse statements include “If x is greater than y, then y is not greater than x,” “If x is not equal to y, then y is not equal to x,” and “If x is less than or equal to y, then y is greater than or equal to x.” It is important to note that the converse of a statement may not be true, even if the original statement is true.
How Does Converse Relate to Logical Statements?
Answer: Converse statements are related to logical statements in that they are statements which can be either true or false, depending on the context. Logical statements are statements which involve logical operations, such as AND, OR, and NOT. For example, “If x is greater than y, then y is not greater than x” is a logical statement.
Why Is It Important to Understand Converse Statements?
Answer: Understanding converse statements is important in mathematics, as it helps us to understand the relationships between different statements, and to determine whether a statement is true or false. It can also help us to identify mistakes in our reasoning and to make sure that we are making sound logical arguments.
What Is the Difference Between Converse and Inverse?
Answer: The difference between converse and inverse is that the converse of a statement is formed by reversing the order of the two parts of the original statement. For example, the converse of the statement “If x is greater than y, then y is less than x” is “If y is less than x, then x is greater than y.” The inverse of a statement, on the other hand, is the negation of the statement. For example, the inverse of the statement “If x is greater than y, then y is less than x” is “If x is not greater than y, then y is not less than x.”
Converse, Inverse, & Contrapositive – Conditional & Biconditional Statements, Logic, Geometry
Math is an important part of our everyday lives, and understanding what converse means can help make the process of solving math problems easier. Converse in math simply means the inverse of the given statement. It’s important to understand the concept of converse in order to be able to understand and solve complex math problems. With the right understanding and practice, converse in math can become second nature.
