Geometry is an essential part of mathematics and is used in many different areas of everyday life. It is also an important subject to understand when it comes to problem-solving. One of the key concepts in geometry is converse, which is a type of statement that is used to describe a relationship between two elements. In this article, we’ll discuss what converse is in geometry and how it is used.
Converse in Geometry is a logical statement that is the inverse of another statement. It is a fundamental property of logic and mathematics, where two statements are related by the fact that the truth of one implies the truth of the other. In geometry, a converse statement is often written in the form of a theorem, which is a statement that can be proven to be true by using certain assumptions or axioms. For example, the theorem “If two angles are congruent, then they are equal” is a converse statement of the statement “If two angles are equal, then they are congruent”.
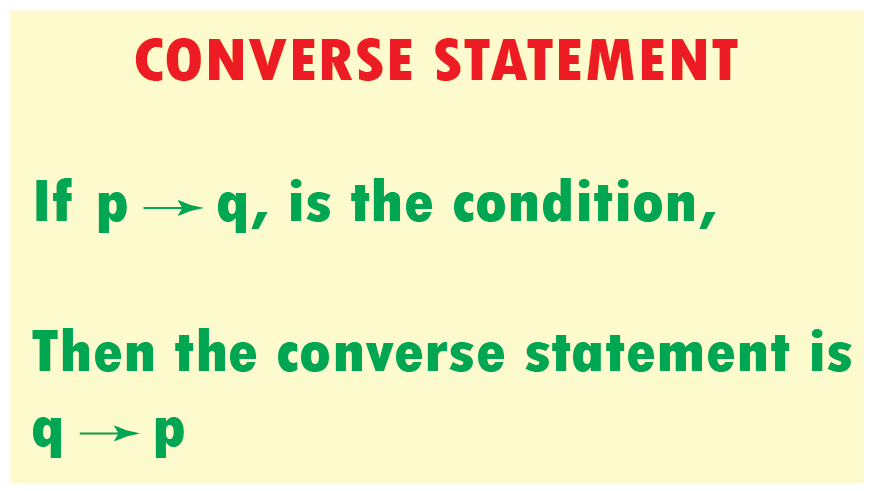
What is Converse in Geometry?
Converse in geometry is a form of logical argument that states that if a statement is true, then the opposite statement must also be true. In the simplest terms, a converse is a statement that reverses the terms of a given proposition. In geometry, the converse of a statement is often used to make conclusions about the given statement. For example, if a statement says that two angles are equal, then the converse of that statement would be that if one angle is greater than the other, then the angles are not equal.
The converse can also be used to prove geometric theorems. In order to prove a theorem, one must first identify the statement, and then prove its converse. For example, if a theorem states that the sum of the interior angles of a triangle is 180°, then the converse of this statement is that if the sum of the interior angles of a triangle is not 180°, then the triangle is not a triangle.
Examples of Converse in Geometry
One of the most common examples of converse in geometry is the Pythagorean theorem. The Pythagorean theorem states that a² + b² = c², where a and b are the two legs of a right triangle, and c is the hypotenuse. The converse of this statement is that if a² + b² ≠ c², then the triangle is not a right triangle. This converse can also be used to prove that a triangle is a right triangle if the lengths of the legs and the hypotenuse are known.
The converse can also be used to prove theorems about parallel lines. For example, the theorem states that if two lines are parallel, then their corresponding angles are equal. The converse of this statement is that if two lines are not parallel, then their corresponding angles are not equal. This converse can be used to prove that two lines are parallel if their corresponding angles are known.
Uses of Converse in Geometry
The converse of a statement can be used to prove theorems in geometry. By proving the converse of a statement, one can prove that the statement is true. This is because the converse of a statement is equivalent to the statement itself, so if the converse is proven to be true, then the statement must also be true.
Converse can also be used to solve geometry problems. By identifying the statement and its converse, one can identify which statement is true and which is false. From there, one can use the true statement to solve the problem.
What is the Difference Between Converse and Contrapositive?
The converse of a statement is the statement with the terms reversed, while the contrapositive is the statement with the terms both reversed and negated. For example, if a statement is “If A, then B,” the converse would be “If B, then A,” and the contrapositive would be “If not B, then not A.”
Difference in Meaning
The converse of a statement does not necessarily mean the same thing as the original statement, whereas the contrapositive does. For example, if the statement is “If A, then B,” the converse is “If B, then A,” but this does not necessarily mean that A and B are related. The contrapositive, however, states “If not B, then not A,” which implies that A and B are related in some way.
Difference in Proving Theorems
The converse and the contrapositive can both be used to prove theorems, but they are used in different ways. The converse is usually used as a starting point to prove a theorem, while the contrapositive is usually used to prove the converse. For example, if one wants to prove that two lines are parallel, one might start by proving the converse statement that if the lines are not parallel, then their corresponding angles are not equal. Then, one can use the contrapositive to prove that if the corresponding angles are equal, then the lines must be parallel.
Difference in Logic
The converse of a statement is logically equivalent to the original statement, whereas the contrapositive is logically equivalent to the inverse of the original statement. For example, if the statement is “If A, then B,” the converse is logically equivalent to the original statement, but the contrapositive is logically equivalent to the statement “If not A, then not B.”
Few Frequently Asked Questions
What is Converse in Geometry?
Answer: Converse in geometry is the process of switching two statements around to form a new statement. Specifically, it involves making the hypothesis the conclusion and vice versa. This process is used to prove or disprove a theorem, by showing that if a statement’s converse is false, then the original statement must also be false.
How is Converse used in Geometry?
Answer: Converse is commonly used in geometry to prove or disprove a theorem or statement. To prove a statement true, the converse must also be proven true, while to disprove the statement, it is necessary to prove the converse false. To do this, a proof is constructed which shows that if the converse of the statement is false, then the original statement must also be false.
What is the Relationship between Converse and Contrapositive?
Answer: The relationship between converse and contrapositive is that the contrapositive of a statement is the converse of the statement’s inverse. A statement’s inverse is simply the statement with the hypothesis and conclusion reversed. Therefore, the contrapositive of a statement is the converse of the statement with the hypothesis and conclusion reversed.
What is an Example of a Converse Statement?
Answer: An example of a converse statement is: “If two lines intersect, then they are not parallel”. This statement is the converse of the original statement: “If two lines are not parallel, then they intersect”. By switching the hypothesis and conclusion of the statement, the converse is formed.
What is an Example of Proving a Converse Statement?
Answer: An example of proving a converse statement is the following proof: Given: Two lines that intersect. Prove: The lines are not parallel.
Proof: Assume the lines are parallel. However, by the definition of parallel lines, they would not intersect. This is a contradiction, so the assumption that the lines are parallel must be false. Therefore, the lines are not parallel.
What are the Benefits of Using Converse in Geometry?
Answer: The main benefit of using converse in geometry is that it allows for the proof or disproval of a theorem or statement. By switching the hypothesis and conclusion of a statement, a new statement is formed which can be used to prove or disprove the original statement. This process is particularly useful in proving theorems, as it can provide a logical argument which is backed up by facts and evidence.
What is the Corresponding Angle Converse Theorem
In conclusion, converse in geometry refers to the relationship between the hypothesis and the conclusion of a statement. A converse statement is formed by switching the hypothesis and conclusion of a logical statement. It is important to understand the concept of converse in geometry in order to make logical deductions and solve geometric problems. With a basic understanding of how to apply converse in geometry, students can confidently approach any geometry problem they come across.
