Math can be a daunting subject, but understanding the basics of converse in math can give you a great foundation to build your mathematical skills. In this article, we’ll look at what converse in math is and how it can help you understand more complex mathematical concepts. We’ll also discuss how you can use converse in math to solve problems and make predictions. By the end, you should have a clear understanding of what converse in math is and how it can help you succeed in your math classes.
What is Converse in Math?
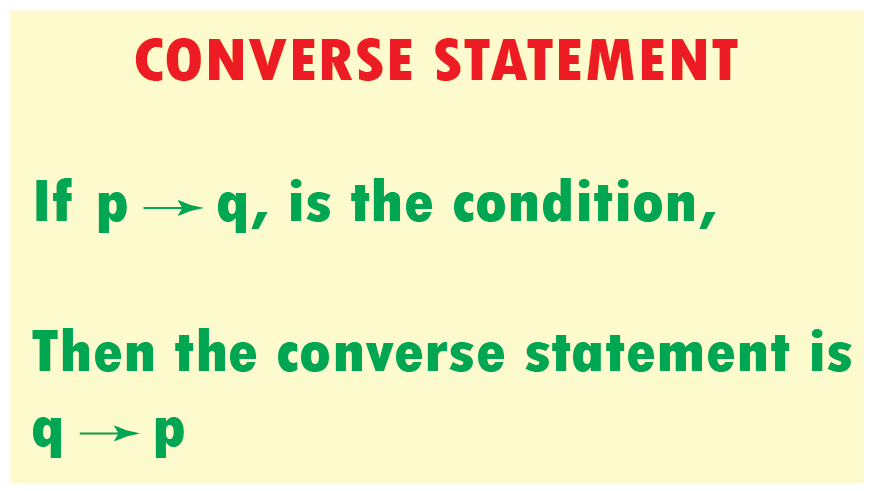
Converse in math is a method of switching the terms of a statement. It is used to help determine whether a statement is true or false by reversing the terms. It is an important part of logical reasoning and can be applied to many different types of problems. The converse of a statement is the inverse of the original statement, so it is important to understand the converse of a statement before attempting to use it.
Identifying the Converse of a Statement
The converse of a statement is found by switching the two terms of the original statement. For example, the statement “If it rains, then the grass is wet” can be reversed by switching the terms to “If the grass is wet, then it rains.” Once the terms are switched, the converse of the statement is created.
In order to determine whether the converse of a statement is true or false, one must consider the conditions of the original statement. If the original statement is true, then the converse of the statement is also true. However, if the original statement is false, then the converse of the statement may not be true.
Using the Converse to Solve Problems
The converse of a statement can be used to solve many different types of problems. For example, if one is given a set of data and is asked to determine whether a statement is true or false, they can use the converse to help them answer the question. By reversing the terms of the statement, one can use the data to determine whether the original statement is true or false.
The converse can also be used to prove the validity of a statement. By using the converse of a statement, one can prove that a statement is true by showing that its converse is also true. This is because if the converse is true, then the original statement must also be true.
Examples of Converse in Math
The converse of the statement “If it is raining, then the grass is wet” is “If the grass is wet, then it is raining.” This converse can be used to prove that the original statement is true. For example, if one were to observe that the grass is wet, then they can conclude that it must have been raining.
The converse of the statement “If a number is odd, then it is not divisible by 2” is “If a number is not divisible by 2, then it is odd.” This converse can be used to prove that the original statement is true. For example, if one were to observe that a number is not divisible by 2, then they can conclude that the number must be odd.
Application of Converse in Math
The converse of a statement can be used to prove the validity of a statement, as well as to solve many types of problems. It can also be used to determine whether a statement is true or false by switching the terms of the statement. By understanding the converse of a statement, one can gain a better understanding of the logic behind a problem and be able to solve it more effectively.
Few Frequently Asked Questions
What is Converse in Math?
Answer: Converse in math is a logical statement that is formed by switching the order of the hypothesis and conclusion of a conditional statement. In a conditional statement, the hypothesis is the part that comes first and the conclusion is the part that comes second. For example, if the original statement was “If it rains, then the ground will be wet”, then the converse of this statement would be “If the ground is wet, then it has rained”.
What is an Example of a Converse Statement in Math?
Answer: An example of a converse statement in math is “If the ground is wet, then it has rained”. This statement is formed by switching the order of the hypothesis and conclusion of the original statement, which was “If it rains, then the ground will be wet”.
What is the Difference Between a Converse and a Contrapositive?
Answer: The difference between a converse and a contrapositive statement in math is that a converse statement switches the order of the hypothesis and conclusion of a conditional statement, while a contrapositive statement switches the order of the hypothesis and conclusion and then negates both parts of the statement. For example, the converse of “If it rains, then the ground will be wet” would be “If the ground is wet, then it has rained”, while the contrapositive of the same statement would be “If the ground is not wet, then it has not rained”.
When Should a Converse Statement be Used?
Answer: A converse statement in math should be used when trying to infer information from a given condition. For example, if a person wanted to know if it has rained, they could look at the ground to see if it is wet. If it is wet, then they could infer that it has rained, using the converse statement “If the ground is wet, then it has rained”.
Are Converse Statements Always True?
Answer: No, converse statements are not always true. This is because a converse statement is formed by switching the order of the hypothesis and conclusion of a conditional statement, which can change the truth value of the statement. For example, the converse of “If it is raining, then the ground will be wet” is “If the ground is wet, then it is raining”. While this statement is true in some situations, it is not always true, as the ground can be wet for other reasons besides rain.
How Can a Person Determine if a Converse Statement is True?
Answer: To determine if a converse statement is true, a person should look at the original conditional statement to see if the converse statement can be logically inferred from the original statement. For example, if the original statement is “If it is raining, then the ground will be wet”, then the converse statement “If the ground is wet, then it is raining” can be logically inferred from this statement and is thus true. However, if the original statement was “If it is sunny, then the ground will be wet”, then the converse statement “If the ground is wet, then it is sunny” would not be true, as the ground can be wet for other reasons besides sun.
Converse in math is an important concept for understanding how arguments and logic work. It is used to examine the relationship between two statements and determine if the opposite of one of the statements can be logically concluded. By understanding converse in math, students can gain a better understanding of logical deduction and how to use it to solve problems. With this knowledge, students can use their understanding of converse in math to make informed decisions, solve complex problems and gain a deeper understanding of the world around them.
