Geometry is a branch of mathematics that deals with shapes, sizes, and spatial relationships. It’s essential for anyone who wants to understand how the world works. One of the most important concepts in geometry is the converse, which is a statement that can be used to determine the validity of a given statement. In this article, we’ll explore what the converse in geometry is and how it can help us make better decisions. So, if you’re looking to increase your understanding of the world around you, keep reading to learn more about the converse in geometry.
The converse of a statement is not necessarily true, however. For example, if the statement “If two lines are parallel, then they do not intersect” is true, then its converse, “If two lines do not intersect, then they are parallel,” is not necessarily true; it is possible for two lines to be skew and not intersect.
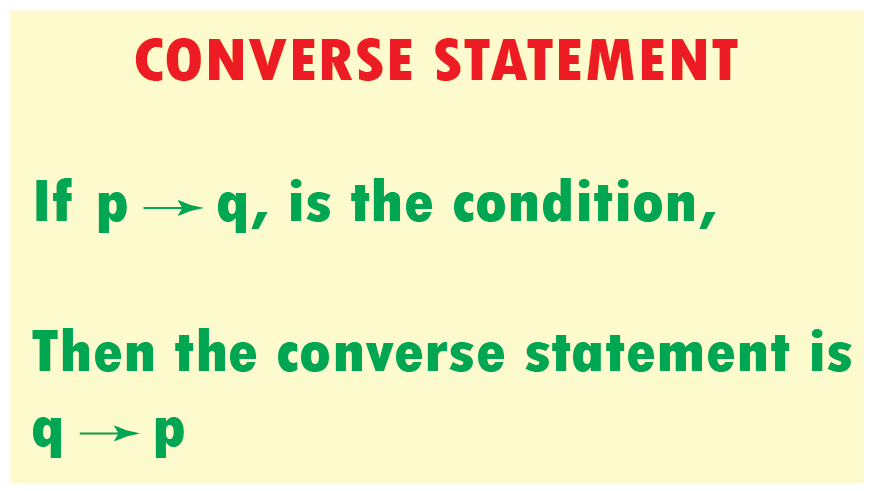
What is Converse in Geometry?
The converse in geometry is a logical statement that is the opposite of a given statement. It is used to determine if a statement is true or false. In geometry, the converse of a statement is a statement that is the opposite of the original statement. For example, the converse of “If two lines are parallel, then they are the same length” would be “If two lines are the same length, then they are parallel.”
In geometry, the converse of a statement is often used to determine the truth of a statement. For example, if a statement is true, then its converse must also be true. For example, if “If two lines are parallel, then they are the same length” is true, then its converse “If two lines are the same length, then they are parallel” must also be true.
The converse of a statement is often used to prove the truth of a statement. For example, if a statement is true, then its converse must also be true in order to prove the truth of the statement. For example, if “If two lines are parallel, then they are the same length” is true, then its converse “If two lines are the same length, then they are parallel” must also be true in order to prove the truth of the statement.
Examples of Converse in Geometry
The converse in geometry is often used to prove the truth of a statement. A few examples of converse in geometry include:
If two angles are equal, then their sides are equal: The converse of this statement is “If two sides are equal, then the angles are equal”.
If two lines are perpendicular, then they intersect at a right angle: The converse of this statement is “If two lines intersect at a right angle, then they are perpendicular”.
If two lines are parallel, then they have the same slope: The converse of this statement is “If two lines have the same slope, then they are parallel”.
Different Types of Converse in Geometry
There are two types of converse in geometry: direct and indirect. A direct converse is a statement that is the opposite of the original statement. An indirect converse is a statement that is the opposite of the conclusion of the original statement.
Direct Converse
A direct converse is a statement that is the opposite of the original statement. For example, the converse of “If two lines are parallel, then they are the same length” is “If two lines are the same length, then they are parallel”.
Indirect Converse
An indirect converse is a statement that is the opposite of the conclusion of the original statement. For example, the converse of “If two lines are the same length, then they are parallel” is “If two lines are not parallel, then they are not the same length”.
Using the Converse in Geometry
The converse in geometry is often used to prove the truth of a statement. To prove the truth of a statement using the converse, the statement must be true, and its converse must also be true. For example, if “If two lines are parallel, then they are the same length” is true, then its converse “If two lines are the same length, then they are parallel” must also be true in order to prove the truth of the statement.
The converse in geometry can also be used to determine if a statement is true or false. If the statement and its converse are both true, then the statement is true. If the statement or its converse is false, then the statement is false.
Related Faq
What is the Converse in Geometry?
The converse in geometry is a statement that is implied by the original statement, but is written in the opposite direction. For example, if the original statement is “If two lines are perpendicular, then they intersect,” then the converse would be “If two lines intersect, then they are perpendicular.” In geometry, the converse of a theorem is not always true, so it must be tested using a counterexample or a proof.
How is the Converse different from the Original Statement?
The converse of a statement is different from the original statement in that it is written in the opposite direction. For example, if the original statement is “If two lines are perpendicular, then they intersect,” then the converse would be “If two lines intersect, then they are perpendicular.” In addition, the converse of a theorem is not always true, whereas the original statement is always true.
What is a Counterexample?
A counterexample is an example that disproves a statement. For example, if the statement is “If two lines are perpendicular, then they intersect,” then a counterexample would be two parallel lines that do not intersect. By providing a counterexample, you can prove that the converse of a theorem is false.
What is the Purpose of a Proof?
A proof is a valid argument that provides evidence of the truth of a statement. For example, if the statement is “If two lines are perpendicular, then they intersect,” then a proof might include a diagram of two perpendicular lines that intersect, along with a valid argument that explains why the lines must intersect. By providing a proof, you can demonstrate that the converse of a theorem is true.
What is an Implication?
An implication is a statement that is implied by another statement. For example, if the statement is “If two lines are perpendicular, then they intersect,” then an implication of this statement would be that if two lines do not intersect, then they are not perpendicular. Implications can be used to identify the converse of a theorem.
Why is it Important to Test the Converse of a Theorem?
It is important to test the converse of a theorem because the converse is not always true. For example, if the statement is “If two lines are perpendicular, then they intersect,” then the converse would be “If two lines intersect, then they are perpendicular.” However, this is not always true, as two parallel lines can intersect each other without being perpendicular. Therefore, it is important to test the converse of a theorem in order to ensure that it is true.
Converse, Inverse, & Contrapositive – Conditional & Biconditional Statements, Logic, Geometry
In conclusion, the converse in geometry is a statement that is the opposite of a given geometric theorem. This statement can be used to prove the original theorem, as well as to develop more advanced theorems. By understanding the concept of converse, students can deepen their understanding of geometry and use it to solve more complex problems.
