Math is a universal language that allows us to explain the world around us and explore the unknown. One of the most important concepts in mathematics is the converse, which is a statement formed by reversing the order of the two statements in a given mathematical proposition. In this article, we will explore what the converse means in math and how it is used to make mathematical statements. So, let’s dive in and discover the converse in math!
The converse in math is the statement that results from switching the hypothesis and conclusion of a conditional statement. In other words, the converse of the statement “If p then q” is “If q then p.” Here, p and q are logical statements. In math, two statements can be either equivalent or independent.
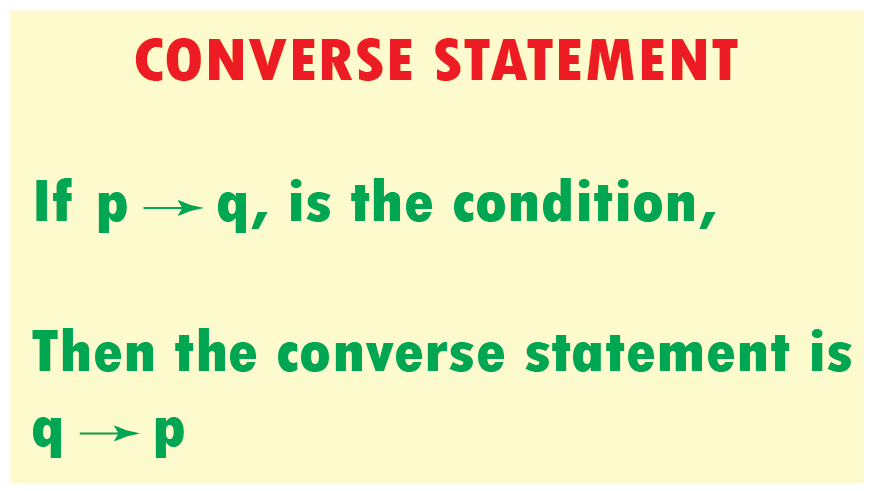
What is the Converse in Mathematics?
Converse in mathematics is a statement that is reversed from the original statement. It is used to determine whether the original statement is true or false. It is often used in mathematical proofs and reasoning to determine the validity of a statement. In other words, the converse of a statement is the opposite of the statement. For example, if a statement is true, the converse of it is false.
How is the Converse Used?
The converse of a statement can be used to prove the validity of a statement by working backwards. This is done by reversing the original statement and determining its truth value. If the converse is true, then the original statement is also true. For example, if the statement “All triangles have three sides” is true, then its converse “All three-sided shapes are triangles” is also true.
The converse can also be used to make predictions. For example, if the statement “All dogs bark” is true, then its converse “All things that bark are dogs” can be used to predict that a barking animal is a dog.
What is the Difference Between Converse and Contrapositive?
The converse of a statement is the reversed version of the statement, while the contrapositive is the statement with the opposite logical relationship. For example, if the statement is “If it is raining, then the ground is wet”, then its converse is “If the ground is wet, then it is raining” and its contrapositive is “If the ground is not wet, then it is not raining”.
Examples of Converses
The converse of the statement “If it is cold, then the windows are closed” is “If the windows are closed, then it is cold”.
The converse of the statement “If it is a triangle, then it has three sides” is “If it has three sides, then it is a triangle”.
The converse of the statement “If it is a square, then it has four sides” is “If it has four sides, then it is a square”.
How to Prove a Converse Statement
In order to prove a converse statement, one must first prove that the original statement is true. Once the original statement is proven to be true, then the converse statement can be proven by reversing the statement and using the same proof. For example, if the original statement is “If it is a triangle, then it has three sides” and it has been proven to be true, then the converse statement “If it has three sides, then it is a triangle” can be proven by reversing the statement and using the same proof.
Using Converses in Mathematical Proofs
Converses can be used in mathematical proofs to prove the validity of a statement. A statement and its converse can be used together to prove that a statement is true. For example, if the statement is “If it is a triangle, then it has three sides” and its converse “If it has three sides, then it is a triangle” are both proven to be true, then it can be concluded that the statement is true.
Related Faq
What is the Converse in Math?
Answer: The converse in math is when you take a statement and switch the two parts of the statement. In a mathematical statement, the converse is usually the logical inverse of the given statement. For example, if the statement is “if x is greater than y, then y is less than x,” the converse would be “if y is less than x, then x is greater than y.”
What is the Difference between the Converse and the Inverse?
Answer: The difference between the converse and the inverse is that the converse switches the two parts of a statement while the inverse negates both parts of the statement. For example, if the statement is “if x is greater than y, then y is less than x,” the converse would be “if y is less than x, then x is greater than y” while the inverse would be “if x is not greater than y, then y is not less than x.”
How Do You Find the Converse of a Statement?
Answer: To find the converse of a statement, you need to switch the two parts of the statement. For example, if the statement is “if x is greater than y, then y is less than x,” the converse would be “if y is less than x, then x is greater than y.”
What is an Example of the Converse in Math?
Answer: An example of the converse in math is “if x is greater than y, then y is less than x,” and its converse would be “if y is less than x, then x is greater than y.”
How Can You Use the Converse in Math?
Answer: The converse can be used in math to prove statements or to determine whether a statement is true or false. For example, if you know that “if x is greater than y, then y is less than x” is true, then you can use the converse to prove that “if y is less than x, then x is greater than y” is also true.
What Does the Converse Tell Us?
Answer: The converse tells us that when the two parts of a statement are switched, the statement stays true. For example, if the statement “if x is greater than y, then y is less than x” is true, then its converse “if y is less than x, then x is greater than y” is also true. This is because the converse is the logical inverse of the statement.
The converse in math is a type of statement that is formed by swapping the two components of a conditional statement. It is an important concept to understand, as it can be used to prove the validity of certain assumptions and make deductions. In essence, the converse in math is an essential tool for problem solving and logical reasoning. With a clear understanding of the converse in math, you can better equip yourself to tackle complex problems and arrive at the most accurate solutions.
