The Corresponding Angles Theorem is one of the most fundamental theorems in geometry. It states that if two parallel lines are cut by a transversal, then the corresponding angles formed are congruent. But what is the converse of this theorem? In other words, how do we determine if two angles are congruent if we know nothing about the lines they are on? In this article, we will explore the answer to this question and examine the implications of the Converse of the Corresponding Angles Theorem.
The Converse of the Corresponding Angles Theorem states that if two lines are cut by a transversal and the corresponding angles are congruent, then the two lines are parallel.
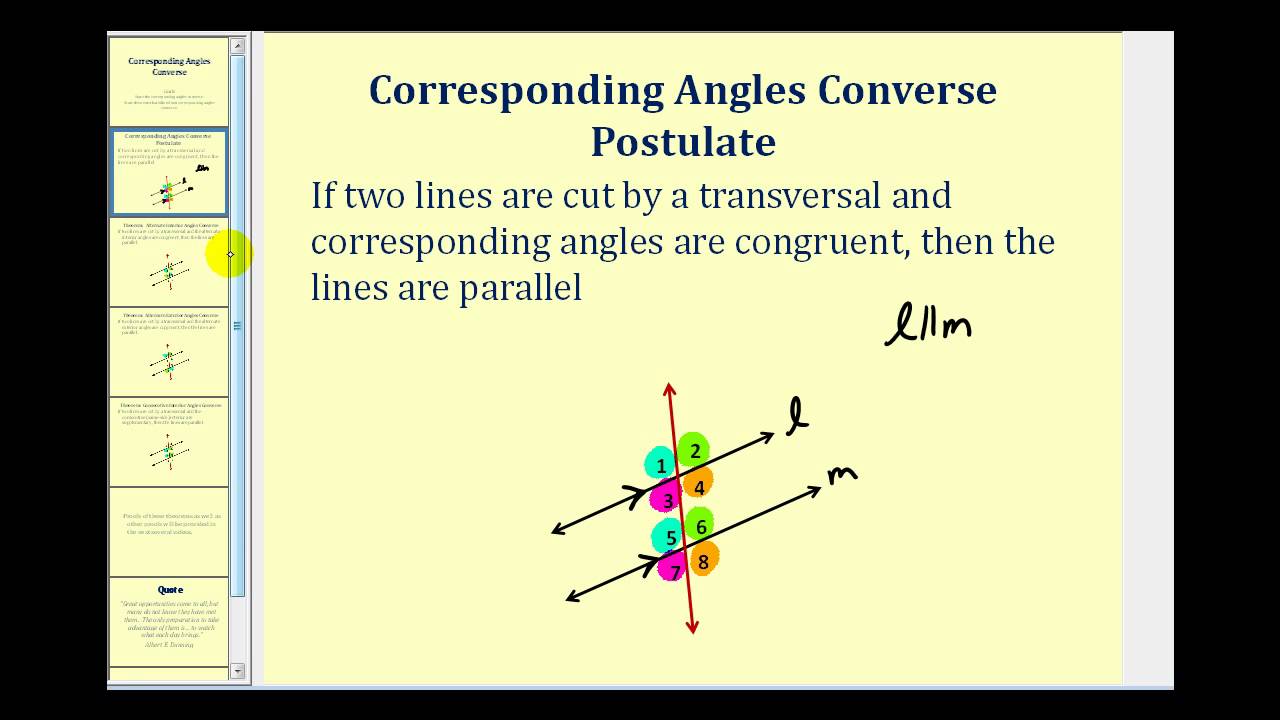
The Converse of the Corresponding Angles Theorem Explained
The Converse of the Corresponding Angles Theorem states that if two lines are cut by a transversal and the corresponding angles are equal, then the two lines are parallel. This theorem is the converse of the Corresponding Angles Theorem which states that if two lines are parallel, then the corresponding angles are equal. This theorem can be used to prove that two lines are parallel and can be used as a tool to build strong proofs.
The Converse of the Corresponding Angles Theorem can be stated as follows: Given two lines cut by a transversal, if the corresponding angles are equal, then the two lines are parallel. This theorem is a direct consequence of the Corresponding Angles Theorem, which states that if two lines are parallel, then the corresponding angles are equal. This theorem can be used to prove that two lines are parallel and can be used as a tool to build strong proofs.
The Converse of the Corresponding Angles Theorem is a very powerful tool when it comes to proving that two lines are parallel. By using this theorem, it can be determined that two lines are parallel without having to directly measure the angles or draw the lines. This theorem can also be used to prove that two lines are not parallel, by showing that the corresponding angles are not equal.
Proof of the Converse of the Corresponding Angles Theorem
In order to prove the Converse of the Corresponding Angles Theorem, it is necessary to use the principles of geometry. The first step is to draw two lines and a transversal that cuts across both lines. The next step is to measure the angles formed by the transversal and the two lines. If the corresponding angles are equal, then the two lines are parallel.
To prove the theorem, it is necessary to use the definition of parallel lines. Two lines are parallel if they do not intersect. This implies that the angle between the two lines is equal to 0. If the corresponding angles are equal, then it follows that the angle between the two lines is equal to 0. Thus, the two lines are parallel.
Example of the Converse of the Corresponding Angles Theorem
The following example illustrates the Converse of the Corresponding Angles Theorem. Consider two lines and a transversal that cuts across both lines. If the corresponding angles are equal, then the two lines are parallel.
Case 1
In this case, the corresponding angles are equal. This implies that the angle between the two lines is equal to 0. Thus, the two lines are parallel.
Case 2
In this case, the corresponding angles are not equal. This implies that the angle between the two lines is not equal to 0. Thus, the two lines are not parallel.
Applications of the Converse of the Corresponding Angles Theorem
The Converse of the Corresponding Angles Theorem can be used in many different areas of mathematics, such as geometry, trigonometry, and calculus. In geometry, this theorem can be used to prove that two lines are parallel without having to directly measure the angles or draw the lines. In trigonometry, this theorem can be used to prove various relationships between angles. In calculus, this theorem can be used to prove various theorems related to derivatives and integrals.
Geometry
In geometry, the Converse of the Corresponding Angles Theorem can be used to prove that two lines are parallel without having to directly measure the angles or draw the lines. This theorem can also be used to prove that two lines are not parallel, by showing that the corresponding angles are not equal.
Trigonometry
In trigonometry, this theorem can be used to prove various relationships between angles. For example, the theorem can be used to prove the Law of Sines, which states that the ratios of the sides of a triangle are equal to the ratios of the sines of the opposite angles.
Calculus
In calculus, this theorem can be used to prove various theorems related to derivatives and integrals. For example, the theorem can be used to prove the Mean Value Theorem, which states that the average value of a continuous function over a closed interval is equal to the value of the function at some point in the interval.
Top 6 Frequently Asked Questions
What is the Converse of the Corresponding Angles Theorem?
The converse of the corresponding angles theorem states that if two parallel lines are cut by a transversal, then the corresponding angles are equal. This theorem is the converse of the corresponding angles theorem, which states that if two lines are cut by a transversal and the corresponding angles are equal, then the lines are parallel. Thus, the converse of the corresponding angles theorem can be expressed mathematically as: ‘If ∠1 = ∠2, then lines l and m are parallel.’
What are the conditions for the Converse of the Corresponding Angles Theorem?
The converse of the corresponding angles theorem requires two conditions to be fulfilled. Firstly, two lines must be cut by a transversal. Secondly, the corresponding angles must be equal. This means that the angles opposite each other and on the same side of the transversal must be equal. If these two conditions are satisfied, then the lines are parallel.
Are the angles opposite each other on the same side of the transversal?
Yes, the angles opposite each other on the same side of the transversal must be equal in order for the converse of the corresponding angles theorem to be true. This means that the angles on one side of the transversal must be the same as the angles on the other side of the transversal.
What is the mathematical expression for the Converse of the Corresponding Angles Theorem?
The mathematical expression for the converse of the corresponding angles theorem is: ‘If ∠1 = ∠2, then lines l and m are parallel.’ This expression states that if two lines are cut by a transversal and the corresponding angles are equal, then the lines are parallel.
What is the relationship between the Corresponding Angles Theorem and its Converse?
The corresponding angles theorem and its converse are related in that they both involve two lines cut by a transversal. The corresponding angles theorem states that if two parallel lines are cut by a transversal, then the corresponding angles are equal. The converse of the corresponding angles theorem states that if the corresponding angles are equal, then the lines are parallel.
What is the significance of the Converse of the Corresponding Angles Theorem?
The converse of the corresponding angles theorem is significant because it allows us to determine whether two lines are parallel or not. This makes it a valuable tool for solving problems in geometry, as it allows us to determine whether two lines are parallel without having to measure the angles. This makes the converse of the corresponding angles theorem a useful tool in problem solving and in mathematics more generally.
What is the Corresponding Angle Converse Theorem
The Converse of the Corresponding Angles Theorem states that if two lines are cut by a transversal and the corresponding angles are congruent, then the two lines must be parallel. This theorem is an essential tool used in geometry, and it is important to remember when studying the subject. By understanding this theorem and its converse, we can better understand the relationship between parallel lines, congruent angles, and transversals.
